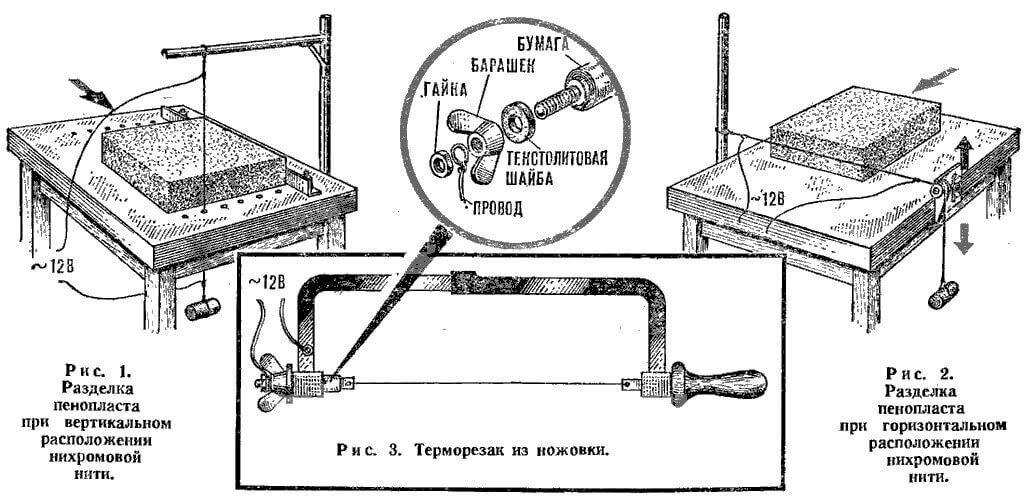
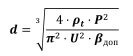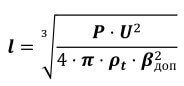How to calculate the length of a nichrome wire

Basic information and brands of nichrome

Nickel is an alloy of nickel and chromium with the addition of manganese, silicon, iron, aluminum. For this material, the parameters depend on the specific ratio of substances in the alloy, but on average lie in the range:

• electrical resistivity - 1.05-1.4 Ohm * mm²/ m (depending on the grade of alloy);
• temperature coefficient of resistance - (0.1-0.25) · 10⁻³ TO⁻¹;
• operating temperature - 1100 ° C;
• melting point - 1400 ° C;

In the tables, resistivity is often given in μΩ * m (or 10^-6 Ohm * m) - the numerical values ​​are the same, the difference in dimension.

There are currently two of the most common brands of nichrome wire:

• X20H80. It consists of 74% nickel and 23% chromium, as well as 1% iron, silicon and manganese. Conductors of this brand can be used at temperatures up to 1250 ^ᵒC, melting point - 1400 ^ᵒC. It also has a high electrical resistance. The alloy is used for the manufacture of elements of heating devices. The specific resistance is 1.03-1.18 μOhm · m;
• X15H60. Composition: 60% nickel, 25% iron, 15% chromium. Operating temperature no more than 1150 ^ᵒC. Melting point - 1390 ^ᵒC. It contains more iron, which increases the magnetic properties of the alloy and increases its corrosion resistance.

You will learn more about the grades and properties of these alloys from GOST 10994-74, GOST 8803-89, GOST 12766.1-90 and others.

As already mentioned, nichrome wire is used everywhere where heating elements are needed. High resistivity and melting point make it possible to use nichrome as the basis for various heating elements, from a kettle or hairdryer to a muffle furnace.

Calculation Methods

By resistance

Let's figure out how to calculate the length of a nichrome wire in terms of power and resistance. The calculation begins with determining the required power. Imagine that we need a nichrome thread for a small soldering iron with a power of 10 watts, which will work from a 12V power supply. To do this, we have a wire with a diameter of 0.12 mm.

The simplest calculation of nichrome length in power without taking into account heating is performed as follows:

We determine the current strength:

P = UI

I = P / U = 10/12 = 0.83 A

Calculation of the resistance of a nichrome wire is carried out by Ohm's law:

R = U / I = 12 / 0.83 = 14.5 Ohm

The length of the wire is:

l = SR /ρ,

where S is the cross-sectional area, ρ – resistivity.

Or according to this formula:

l = (Rπd2) / 4ρ

But first you need to calculate the resistivity for nichrome wire with a diameter of 0.12 mm. It depends on the diameter - the larger it is, the less resistance.

L = (14.5 * 3.14 * 0.12 ^ 2) / 4 * 1.1 = 0.149m = 14.9cm

The same can be taken from GOST 12766.1-90 table. 8, where a value of 95.6 Ohm / m is indicated, if you count it, you get almost the same thing:

L = R_required/ R_tabl= 14.4 / 95.6 = 0.151m = 15.1cm

For a 10-watt heater that is powered by 12V, you need 15.1cm.

If you need to calculate the number of turns of a spiral to twist it from a nichrome wire of this length, then use the following formulas:

Length of one turn:

l₁= π (D + d / 2),

Number of turns:

N = L / (π (D + d / 2)),

where L and d are the length and diameter of the wire, D is the diameter of the rod on which the spiral will be wound.

Suppose we will wind a nichrome wire onto a rod with a diameter of 3 mm, then the calculations are carried out in millimeters:

N = 151 / (3.14 (3 + 0.12 / 2)) = 15.71 turns

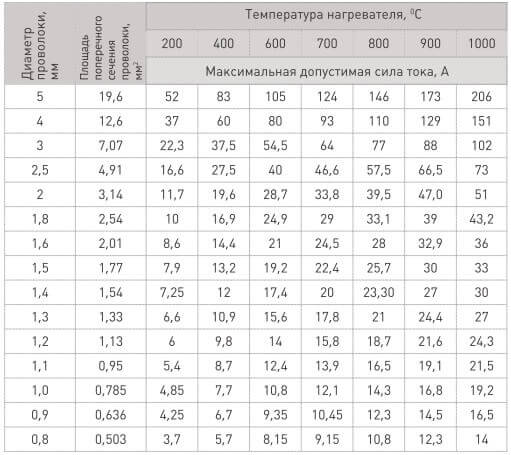
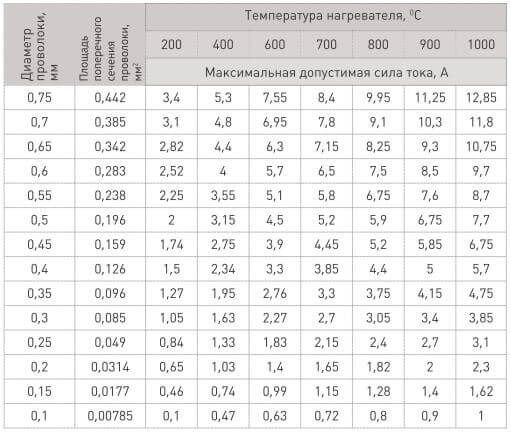
But at the same time, one must take into account whether or not nichrome of such a cross section can withstand this current. Detailed tables for determining the maximum permissible current at a certain temperature for specific sections are given below. In simple words - you determine how many degrees the wire should heat up and select its cross section for the rated current.

Also note that if the heater is inside the liquid, then the current can be increased by 1.2-1.5 times, and if in a confined space, then vice versa - reduced.

By temperature

The problem of the above calculation is that we consider the resistance of a cold spiral along the diameter of the nichrome thread and its length. But it depends on the temperature, while it is also necessary to consider under what conditions it will be possible to achieve it. If this calculation is still applicable for cutting foam or for a heater, then it will be too coarse for a muffle furnace.

We give an example of nichrome calculations for a furnace.

First, determine its volume, let's say 50 liters, then determine the power, for this there is an empirical rule:

• up to 50 liters - 100W / l;
• 100-500 liters - 50-70 W / l.

Then in our case:

P = p_emp* V = 50 * 100 = 5 kW.

Next, we consider the current strength and resistance:

For 220V:

I = 5000/220 = 22.7 Amps

R = 220 / 22.7 = 9.7 Ohms

For 380V when connecting the spirals with a star, the calculation will be as follows.

Divide the power into 3 phases:

Pf = 5/3 = 1.66 kW per phase

When connected by a star, 220V is applied to each branch (phase voltage, it may differ depending on your electrical installation), then the current:

I = 1660/220 = 7.54 A

Resistance:

R = 220 / 7.54 = 29.1 Ohm

For a triangle connection, we calculate according to a linear voltage of 380V:

I = 1660/380 = 4.36 A

R = 380 / 4.36 = 87.1 Ohm

To determine the diameter, the specific surface power of the heater is taken into account. We calculate the length, we take the resistivity from the table. 8. GOST 12766.1-90, but first we define the diameter.

To calculate the specific surface power of the furnace using the formula.

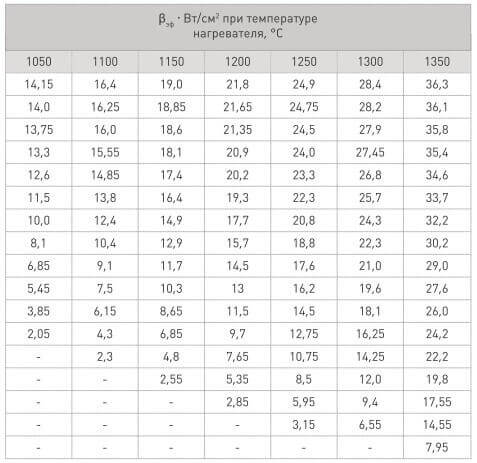
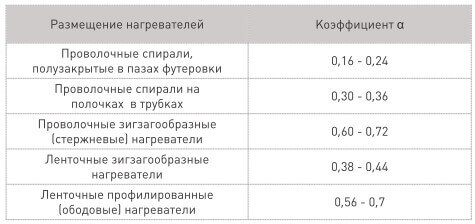
Bet (depends on the heat-absorbing surface) and a (coefficient of radiation efficiency) are selected according to the following tables.

So, to heat the furnace to 1000 degrees, take the spiral temperature of 1100 degrees, then according to the selection table B_ef choose a value of 4.3 W / cm², and according to the selection table of coefficient a - 0.2.

AT_additional= B_ef* a = 4.3 * 0.2 = 0.86 W / cm² = 0.86 * 10 ^ 4 W / m²

The diameter is determined by the formula:

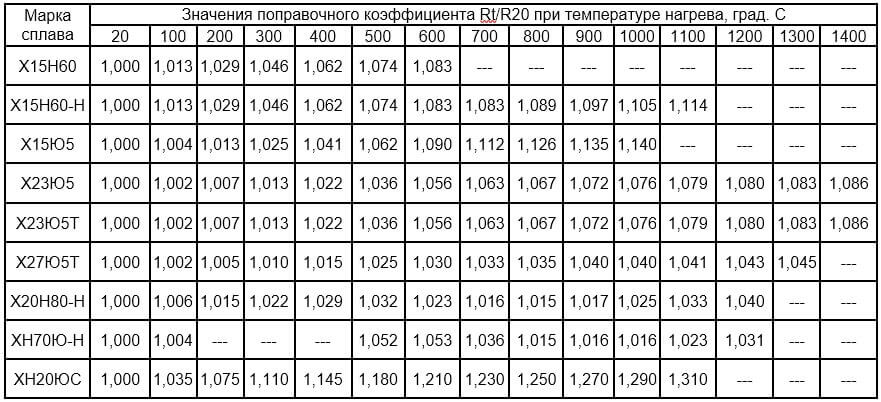
R_t - the specific resistance of the heater material at a given t is determined according to GOST 12766.1, table 9 (shown below).

For nichrome X80N20 - 1,025

R_t= p₂₀*R₁₀₀₀= 1.13 * 10 ^ 6 * 1.025 = 1.15 * 10 ^ 6 Ohm / mm

Then to connect to a three-phase network according to the "Star" scheme:

d = 1.23 mm

The length is calculated by the formula:

L = 42m

Check the values:

L = R / (p * k) = 29.1 / (0.82 * 1.033) = 34m

The values ​​differ due to the high temperature of the spiral; the check does not take into account a number of factors. Therefore, we take for the length of 1 spiral - 42m, then for three spirals you need 126 meters of 1.3 mm nichrome.

Conclusion

Thus, you can calculate the length of the wire for a nichrome spiral and determine the desired diameter by power, cross-section and temperature. It is important to consider:

• environmental conditions;
• location of heating elements;
• spiral temperature;
• temperature to which the surface should be heated and other factors.

Even the above calculation, despite its complexity, cannot be called sufficiently accurate. Because the calculation of heating elements is a continuous thermodynamics, and a number of factors can be cited that influence its results, for example, thermal insulation of a furnace and so on.

In practice, after evaluating the spirals, they add or remove, depending on the result, or use temperature sensors and devices to adjust it.

Related materials:

• How to make an induction boiler yourself
• Calculation of cable cross-section by power and current
• How to calculate wire resistance